Symmetry, Asymmetry, Chirality
Symmetry is an essential concept in as well mathematics and music as in pictorial art. In mathematics it plays such a fundamental role that certain steps in a proof can sometimes be reduced to a simple reference to symmetry. In music and art, however, the use of asymmetry serves to create tension and movement, and in the western tradition, pure symmetry is considered to be static, something to avoid. However, we will take a closer look at two forms of elementary symmetry and how to classify them.
Symmetry & Asymmetry
The mind loves symmetry, perhaps because it is a form of order, of structure, and it has an unquestionable aesthetic appeal. It doesn't exists in nature, however, where an “almost symmetry” is behind everything. This “almost” is everything. With perfect symmetry nothing would exist, matter and antimatter would outbalance and eliminate each other. It is the very small deviation from symmetry that makes existence. In that sense, symmetry is death – or rather, non-existence. Mathematically it is zero.
When we talk about a picture or a pattern, perfect symmetry is not what we mean. It would make the picture disappear. Instead what we refer to is relative symmetry, that is, symmetry in one factor, normally shape or position. The most simple symmetries are based on reflection (in one, two or more axes) respective rotation.
The Difference Between Reflection and Rotation Symmetry
One mirroring (reflection) can switch up and down or left and right, but not both in one step. That means that mirroring is to reflect a picture along one axis, but not two at the same time. However, it is technically possible with a picture to mirror it first in one axis and after that mirror the result in another. If the axes are placed outside of the original picture, with the whole picture entirely included in the reflection, mirroring two times returns us to the original picture, although in a different position - and possibly in a different size.
Let's look at a very simple example.
An axis is a straight line. The red lines below are the axes along which mirroring is done. The original picture is this:

FR can be reflected right-wise like this:

Or downwards like this:

Although it is a form of symmetry, this step is not mirroring, and the bottom picture is the same as the top picture, although rotated. This is rotation symmetry.

You can achieve this by double reflection, two steps of mirroring, creating one of the reflections above and then applying the next on the result.

You can start with FR and reach the picture at bottom right, by first mirroring it horizontally and then vertically, or the other way around. Or you can just turn the original picture upside down instead.
The axes don't have to be perpendicular to lead back to the original picture in two reflections. As long as size, position, or rotation is not a matter of importance, the only requirement is that they are placed outside of the motive that is reflected.
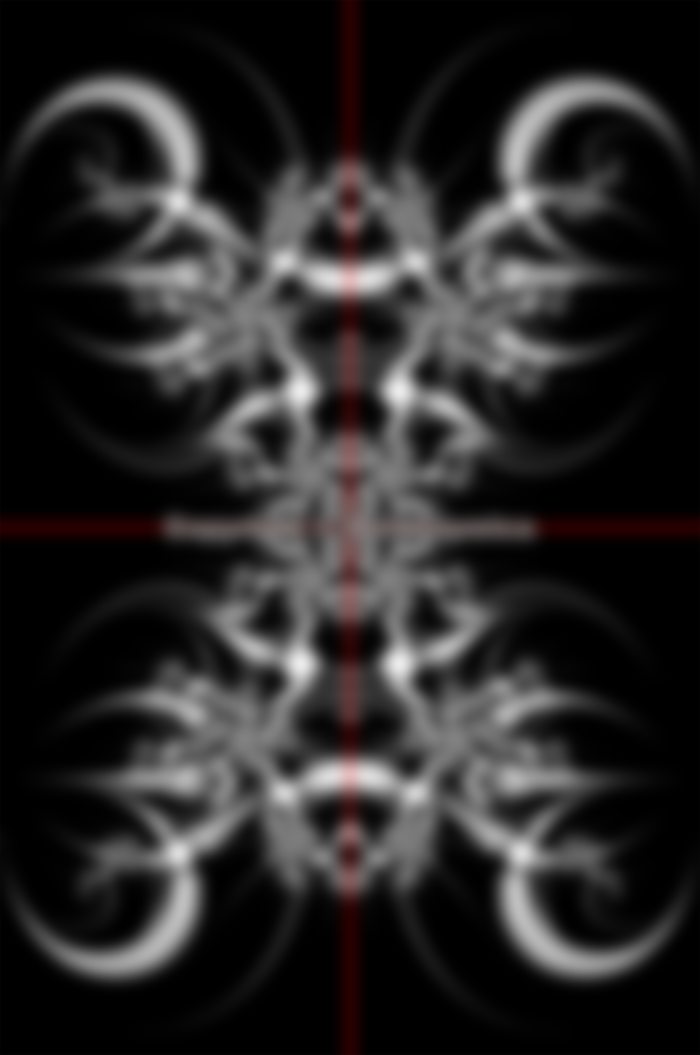
In creating art, however, we do not concern ourselves with axes outside of the pictures, but we apply a method of shaping images by reflection and combination, which moves the axis inside the picture. That means, we combine a picture with its reflection along a straight line, which becomes an axis of symmetry, and get a new picture, after which further modifications may or may not take place. Below is an example of such a picture, where the axis (here marked yellow) is vertical.

Of course we can make two successive reflections in a way that gives us two perpendicular axes of reflection symmetry (here marked in red) through one picture. Here we have both vertical and horizontal reflection symmetry. This is another image based on the same genesis picture, slightly more worked with:

If we look at the next picture (below), we can conclude that the pattern is not created by mirroring. This is rotation symmetry of the second order, and with no reflection. Although that is not how it has been done, it would – at least in theory - be possible to draw an arbitrary axis as a diameter of the circle, through the symmetric centre and create this pattern by copying what's one side of the axis, turn it upside down and combine the two parts along the same axis. This, however, is what we find when we analyse an already existing picture; manually to create one from scratch with that method would be possible, but very difficult when the picture is complex. With mathematics, however, it is possible to define the criteria for such a structure and then we can vary parameters and complexity infinitely until we reach artistically interesting results. This requires too many calculations to be feasible manually, but digitally it is possible.

This is rotation symmetry of the second order, it is bilateral. But the picture below shows trilateral rotation symmetry, it is based on an odd number, and there is no risk for confusion with reflection symmetry.

Chirality
In geometry, chirality is a figure's quality of not being identical to it's mirror image. Such a figure is an enantiomorph. A figure that is not chiral is amphichiral or achiral.
For a figure to be identical to its mirror image, it is required that it can be changed into its mirror image by rotation or simply moving without changing orientation. Every two-dimensional figure that has an axis of reflection symmetry is achiral. Figures or bodies that have rotation symmetry but no reflection symmetry, however, are always chiral.
Being chiral means that they have “handedness”, they are oriented in a certain direction, just as a hand, foot, glove or shoe. You cannot just change the position of a left-foot shoe and make it suit your right foot. That is, the shoe is chiral, it is not identical to its mirror image, because its mirror image is the shoe for the other foot.
The two last pictures above have rotation symmetry but no reflection symmetry. They are chiral, because they possess a “handedness”, they have an orientation, and are not identical to their respective reflections. The two first pictures, however, are achiral. They have no “handedness” and is identical to their reflections.
The concept of chirality is important in science, especially chemistry and crystallography. Molecules and crystals can be with or without chirality, and the distinction is important.
(The article is based on material previously published in Meriondho Leo (2013-2014), and in my e-book “From Vision to Visual Music”, 2017.)
Related articles:
Brain & Horror Vacui (Fear of the void)
Symbols & Their Opposite: Mathematics & Music
Art as Visual Music & The Importance of Form
Practising Art: The Art of Seeing & Beyond Mere Seeing
Copyright © 2013-2014, 2017, 2021 Meleonymica/Mictorrani. All Rights Reserved.
All the images (including the lead image) are mine. Copyright © 2013-2014, 2017, 2022 Meleonymica. All Rights Reserved.
Here you can find my articles about Art, Symbols, Eyes & Vision. and about Philosophy.
Please join my community “Mind, Perception & Thought (c7a5)”.
You find all my writings on Read.Cash, sorted by topic, here.








Hello, my friend
A very interesting article on the mathematical notions of symmetry and asymmetry, as well as, practical considerations in the natural world.
As I read to the end of the article, the curious presence of fractal architecture in nature based on the use of Fibonacci numbers (sequence) came to my mind.
Thank you for sharing.
Best regards.