If you aren't familiar with the game of Plinko, simply put, you first start with a triangle game board that has a bunch of pegs the whole way down. A piece is often dropped at the top center (though this can vary depending on your Plinko game being used) and then the piece works it's way to the bottom of the game board. An example of a Plinko online game board may look like this:

There is some interesting math behind where the pieces will eventually fall, however. As the pieces fall to the bottom, the locations that they will hit are not distributed evenly, far from that as you'll see in a moment. If you'll look on the picture just above, you'll see there are more dice pieces located in the center of the triangle. This is by no means an accident or a coincidence. This is virtual mathematical certainty over time.
On a very simple example, take flipping a coin a single time. You have two outcomes, heads or tails. You can also think of this like a very simple Plinko board where there is just a single peg where the dice can drop to the left or to the right, giving either hole a 50% chance of hitting. But that's not very interesting math or Plinko odds.
If you add in a second set of pegs beneath that first peg, there are now three possible outcomes for your Plinko game. But now things start to get interesting. Because the way they fall down is not a completely even distribution any more. If the first dice falls to the Left, on the next peg it can fall to the Left or to the Right (which would place it in the center). Meanwhile, the dice that initially falls to the Right can go to the Right again or fall to the Left (which would place it in the center.) This can also be written as LL, LR, RL and RR. This ultimately boils down to a 25% chance of the dice going into the far left or far right corners or a 50% chance of it going into the middle section.
Interestingly enough, this can be modeled fairly easily using a system known as Pascal's Triangle. We just calculated row 2 which is seen below. This is done by adding numbers beneath each other as follows:

You'll see the numbers represented on each hexagon. This corresponds to the expected distribution of outcomes of Plinko odds for a given row on a Plinko board. To calculate the numbers on your own, for each hexagon that you see, you simply have to add the higher two numbers to create the number in the present square. You'll see that we calculated from row 2 up above. To get to row 3 for example, you simply have to add the 1 + 2 and 2 + 1 to obtain row 3 equating to 1, 3, 3, 1.
This process can continue indefinitely. It stops at a certain point on the graphic but by following this same pattern, you can keep getting more and more Plinko odds related to the Plinko board of one's choosing. MintDice, for example, uses a Plinko board that ends at row 15. The Plinko odds that MintDice uses are dictated using a Provably Fair algorithm that are then extrapolated onto the roll's outcome which looks like this (which is found on our FAQ):

You'll notice that the big prizes at either end of the Plinko board come with a fairly large multiplier but that they also don't have nearly the same win frequency as some of the other outcomes, such as the common prizes at the middle of the board. Of course, it is worth noting that on the MintDice Plinko game, any prize has the chance to double in value if the dice lands on a six, adding a gamble to the gamble.
The distribution of outcomes on these Plinko boards will tend towards a normal bell shaped curve with many of the outcomes leaning towards the middle of the distribution and falling away from the edges of the distribution. With the nature of a bell shaped curve, one could want to lean more towards consistent outcomes and prizes going for the common wins or one may want to feel lucky and go for the outer edges and gamble hard. These types of distributions can be modeled in theory or in reality. An example is shown below:
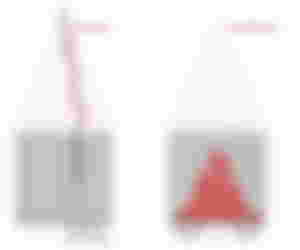
With the knowledge that you have now, it's important to then realize what the options are with the normal Plinko game. If you choose to play on a standard real life wooden board, then you are all set! That kind of thing can be really fun for all kinds of party games or whatever else you dream up. However, if you want to test your luck at playing Plinko online, you will want to make sure first and foremost that you are playing a game that is both provably fair and has a low house edge to maximize your chance of winning. MintDice, of course, has you covered there. As the #1 Bitcoin casino, MintDice has a full suite of bespoke provably fair casino games and skill games to choose from. And of course, with multiple variants of Plinko in both risk of outcome (represented by different colored dice) or themes (doge, for example), you are all set to have some fun with Plinko online.
May the Plinko odds be ever in your favor.
This article was brought to you by the Bitcoin gambling games on MintDice. Originally posted on MintDice.com.



Thank you for sharing your experience. In turn, I want to give you advice too. My argument is that professionals rarely share real secrets with beginners. I went on my search and started with Gembet Singapore. It turned out to be a wise move. I win more often than on other sites and online resources. Now I am thinking of making this my main occupation.